Betty crocheted 5/6 of a blanket, then her cat came along and unraveled 3/5 of what she had done. What part of the whole blanket did the cat undo?
I knew this was a multiplication problem, but it just didn't feel right-- I couldn't see the logic of what was happening. I know "of"-- as in 3/5 "of" 5/6 -- generally translates to multiplication, but my comprehension broke down somewhere between having 5/6 and then talking about the whole blanket. Happily, I have years of experience using Singapore math models, so I knew exactly how to draw it out so I could make it make sense.
One of the strengths of Singapore math is that students use models to work a problem. With these models, even young, concrete-thinking children are able to solve problems that normally require the use of algebra or complicated algorithms.
Here's how it works:
1. Betty crocheted 5/6 of a blanket Draw a bar that represents the blanket and divide it into 6 pieces.
Now identify the part that was crocheted.
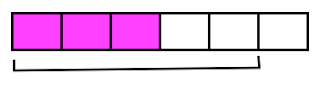
2. ...then her cat came along and unraveled 3/5 of what she had done.
Looking at the bracketed sections, we notice there are five. So 3/5 of the five sections is three sections, which we color in:
3. What part of the whole blanket did the cat undo?
Now it's fairly obvious that the cat unraveled half of the whole blanket.
So, now I can go back to the original problem and see if multiplication gives us the same answer:
Hooray! It does-- 15/30 reduces to 1/2 (or by cross-reducing in the first place, I would have ended up with 1/2 right off.) So multiplication was the way to go, whether it made sense at first or not.
Another example, one that would normally require algebra, might go something like this:
Betty has twice as many cookies as Freya had last night. This morning, Freya ate five of her cookies, but Betty ate none. Now, Betty has four times as many cookies as Freya. How many cookies does Betty have?
So, again, we start with a bar.
We can model the first sentence like this:
Betty has twice as many cookies as Freya had last night.
Here, the top divided bar represents the number of Betty's cookies. The bottom bar represents Freya's cookies. We draw a line in the middle of Betty's bar, exactly in line with the end of Freya's bar, to show that it is twice the value of Freya's.
We can color in Freya's bar or leave it blank.
The next two sentences go together:
This morning, Freya ate five of her cookies, but Betty ate none.
Now, Betty has four times as many cookies as Freya.
We keep Freya's bar the same size, to show what she started with. But now, we use the 1/4 piece of Betty's bar to measure what's left of Freya's cookies. Here, we colored in the remaining value for Freya's cookies. And since we know that Freya ate 5 of her cookies, we know that the difference between the original length of Freya's bar and the new length is 5.
Since we see by the diagram that the 5 cookies turned out to be half of Freya's original amount of cookies, we can fill in each segment with the quantity of 5.
So, Betty has 20 cookies!
To solve this algebraically, we would have done something like this:
Betty has twice as many cookies as Freya had last night. This morning, Freya ate five of her cookies, but Betty ate none. Now, Betty has four times as many cookies as Freya. How many cookies does Betty have?
F = the number of Freya's cookies.
B = the number of betty's cookies
Betty has twice as many cookies as Freya had last night. B = 2F
This morning, Freya ate five of her cookies, but Betty ate none.
Now, Betty has four times as many cookies as Freya. 4(F-5) = B
And solve for F by substitution:
2F = 4(F-5)
2F = 4F - 20
2F-4F = 4F-4F - 20
-2F = -20
-2F/-2 = -20/-2
F = 10
Then solve for B:
B = 2F
B = 2(10)
B = 20
Yeah, I'm liking those math diagrams!
